© Charles Chandler
Having established that 1/3 of the Sun's power is coming from nuclear fusion, and that the other 2/3 is coming from the release of electrostatic potential, we can double-check the synoptic energy budget.
To start, we should make an inventory of the energy that was already contained in the resting dusty plasma, before the collapse began: its initial temperature. On compression, all of that heat will be conserved and concentrated. (Some of it will be lost to EM radiation, but that's a slow process, especially at the cool temperatures during the majority of time the matter is getting compressed, so this article neglects radiative heat loss during the compression. See
Light Curves for a more detailed treatment.) Knowing the elements and their specific heat capacities, we can then estimate the contribution that this energy makes to the total number of joules in the system. (See
this for more info on calculating adiabatic compression.)
First we have to find the pressure of the primordial dusty plasma. The stellar nurseries inside giant molecular clouds are thought to begin with roughly 100 particles per cm3, comprised mostly of diatomic hydrogen, which have a mass of 3.34 × 10−27 kg per molecule. From this we get the density.
The temperature is roughly 10 K.
1,2,3 And we'll go with a molar mass of
2.01 × 10−3 kg/mol (i.e., the same as deuterium). Now we can apply the molar form of the Ideal Gas Law to find the pressure.
Boyle's Law states that in any adiabatic gas, the product of the pressure and the volume is always the same.
The utility of this is that we know the pressure and the volume of the primordial plasma, and we know the volume of the Sun as it is now, and from that, we can find the expected pressure of the Sun.
Then we can find the expected temperature in kelvins using the Combined Gas Law.
First let's find the denominator.
And now we can divide that into the ending PV, to find the ending temperature.
Before we go any further, we should acknowledge the impossibility that these numbers present for the standard model, which has hydrogen fusion sustained by a core temperature of 1.50 × 107 K, and a core pressure of 2.35 × 1016 Pa. Adiabatic compression produces a temperature of 4.47 × 109 K, and a pressure of 2.60 × 1016 Pa, throughout the entire volume of the Sun, not including any internal heat source, nor any pressure from gravity. If the temperatures and pressures in the standard model were sufficient for sustained hydrogen fusion, the far greater temperatures and pressures resulting from adiabatic compression would have caused a supernova. Or more realistically, the temperature and pressure never would have gotten that high — the increasing pressure would have halted the implosion, and initiated a hydrostatic rebound (since gravity is sufficient to contain the Sun's average pressure of 8.98 × 1013 Pa, but insufficient to contain 2.60 × 1016 Pa). So it isn't that a fusion furnace isn't quite necessary, because we already had enough thermodynamic potential (for a supernova!) — it's that we already had two orders of magnitude more pressure than gravity could contain, and we need a fundamentally different organizing principle.
The current-free double-layer (
CFDL) model takes a different approach. The thermal energy of the primordial dusty plasma is incontrovertible, so it has to be taken as a given. This begs the question of where all of that thermal energy went. The answer is that it got converted to electrostatic potential. Under the enormous pressure of implosion, and with the force of gravity thereafter, electron degeneracy pressure (
EDP) separated charges into
CFDLs. The resulting electric fields removed the degrees of freedom, thereby reducing the temperature,
4 down to the 6000 K we observe at the Sun's surface. The heat is recoverable if the electric charges are allowed to recombine, where arc discharges regenerate thermal energy from electrostatic potential. Thus the conservation of energy is maintained throughout the life cycle of the system, but right now, the Sun is deceptively cool, considering the far greater amount of thermal energy that was pumped into it.
Knowing the temperature, we can calculate the joules from the specific heat capacities of the various elements within the Sun.
The Sun has a total mass of 1.99 × 1030 kg, and the standard model asserts that it is 75% hydrogen and 25% helium, with traces of heavier elements. A 75/25 average of the heat capacities of hydrogen & helium is 12026.25 J·kg·K.
But the
Elements section demonstrated the presence of a wide variety of elements in the Sun, and each element has a different specific heat capacity. So it is more accurate to read the
elements table to find the specific heats, and to find the
masses of each element from the properly weighted abundances (using
this code). Those masses can be multiplied by the compacted temperature of
4.47 × 109 K, and by their respective heat capacities, to get the
Joules per element, which can be summed to get the total. The result returned by
this code is
1.11 × 1043 Joules, so that's the number that will be used in subsequent calcs.
To estimate the total energy in the system, we also have to include the momentum of the collapsing dusty plasma that formed the Sun. This is easy to calculate, if we know the mass and the velocity. The mass of the Sun is a known quantity: 1.99 × 1030 kg. Unfortunately, we don't know the velocity of particles in a collapsing dusty plasma, but we can guess at it. Comets approaching the Sun achieve a velocity of roughly 480 km/s. Of course, they don't start from as far away as the extents of the dusty plasma, nor do they keep getting accelerated all of the way to the center of gravity — the centrifugal force in their elliptical orbits limits the acceleration. So we'll figure that cometary velocities are probably shy, and perhaps something like 700 km/s would be more accurate (assuming that gravity is doing the accelerating — which will be revisited later in this article).
To get the total amount of energy, we add the thermal energy to the momentum.
How much of the original energy does the Sun still have left?
Given that the pressure, before the energy was converted to electrostatic potential, was two orders of magnitude greater than gravity could contain, if the potential is released, the pressure will once again be too great, and the neutralized particles will expand outward due to their hydrostatic pressure. This is already in progress, with the result being the heliospheric "bubble" that the Sun is blowing in the interstellar medium. So it's ashes to ashes, and dusty plasmas all of the way back to dusty plasmas in the end.
*12320 And this means that we can calculate how much time the Sun has left, at its current rate of mass loss. To figure this conservatively, we'll go with a high number for the mass loss, which is
5.75 × 109 kg/s.
5:4 (Of course, this doesn't mean that the Sun will still be something worth considering a star until the very end. But there is another utility for this number that will be demonstrated.)
Knowing the time remaining, and the power output rate, we can double-check the energy budget, given that J = W × s. Note that the total power output is
3.86 × 1026 W, but the
Conversions section established that 1/3 of the Sun's power is coming from nuclear fusion, while something like 1/6 of it is from the energy source driving the supergranules, leaving 1/2 of the power (
1.93 × 1026 W) generated by mass loss at the surface. That's the power that we need to use in our calculations, since the nuclear power is the release of a totally different type of potential that we haven't already included in the budget, and the supergranules are also a different story.
This reveals that the previous estimate of
1.16 × 1043 J was three orders of magnitude too low, so clearly, something is missing. The estimate of the thermal energy in the primordial dusty plasma didn't leave much wiggle room. The only other energy source is the momentum. So we can only conclude that E
k has to be three orders of magnitude greater, meaning that the dusty plasma imploded far more rapidly than we figured. We should have expected this, since we estimated the speed at 700 km/s, which was based entirely on gravity. Yet in the
Accretion section, we saw that gravity was insufficient to cause the dusty plasma collapse, given a resting electrostatic
repulsion that was 5× greater than gravity, while Debye sheaths stripped from their dust grains can create an electrostatic
attraction at least three orders of magnitude greater than gravity. So we can expect the kinetic energy to be three orders of magnitude greater than what gravity would have produced, and
6.67 × 1046 J makes sense.
It might be significant that supernovae seem to be capable of releasing in excess of 10
44 J.
6,7 So a total energy store 10
46 J isn't unrealistic.
And we can refigure the speed of the implosion, now that we know the total energy.
It's significant that the new velocity, with an increase of three orders of magnitude, is now 86.38% the speed of light. The electric force is certainly capable of this, especially when acting over a long period of time. Yet at 86.38% c, we can expect the velocity to start to feel the effects of the limit, since acceleration takes more and more energy nearing the speed of light.
Knowing the velocity of the implosion at the end (
2.59 × 108 m/s), and knowing the pressure developed by the adiabatic compression of the dusty plasma (
2.60 × 1016 Pa), we can now calculate the amount of time that it took the implosion to come to a full stop. We know that gravity inside the Sun can contain an average pressure of
8.98 × 1013 Pa, so that's the hydrostatic equilibrium. Any pressure above that is a hydrostatic overshoot from momentum (which is stable in the present model, with the additional electric force between
CFDLs offsetting the pressure). (Since the hydrostatic equilibrium is 3 orders of magnitude less, it doesn't even show up when rounded to 2 significant digits, but the calculations used the full precision.)
To find the total amount of force of this pressure, we just multiply it by the surface area of the Sun.
This is the force that decelerated the imploding dusty plasma. So we divide that by the mass, to find the (negative) acceleration.
Finally, we apply the acceleration to the initial velocity, to find how long it will take to come to a full stop.
And is there any evidence of star-formation events in that amount of time?
Gamma-ray bursts (GRBs) can last from ten milliseconds to several minutes (and sometimes over two hours), usually followed by a longer-lived "afterglow" emitted at longer wavelengths (X-ray, ultraviolet, optical, infrared, microwave and radio).
8,9 This is interesting because we found that the temperatures and pressures ultimately developed in the implosion of a dusty plasma are sufficient for nuclear fusion, which will release gamma-rays. It makes sense that gamma-rays are emitted for something less than the total time to stop for the implosion, since the conditions only achieve criticality near the end. And it makes sense that this is followed by "afterglow" — that's the star that formed. Furthermore, we would ordinarily expect a thermonuclear explosion to obliterate whatever caused it. But the energy released might merely halt the implosion in progress, leaving a "remnant" of the explosion in the center, which we wouldn't expect otherwise.
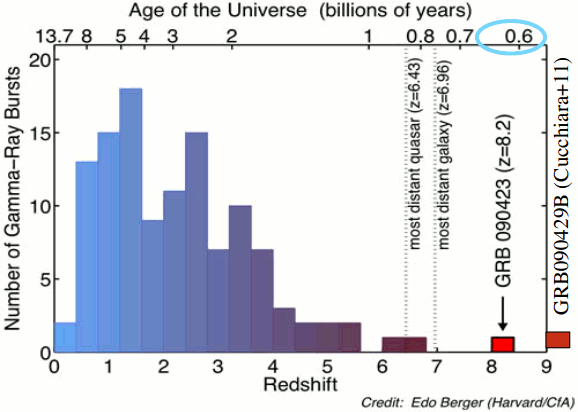
Figure 1.
Distribution of GRBs per redshift.
If GRBs are produced by imploding dusty plasmas, and if the speed can be extreme, they might not be as far away as is conventionally believed. GRBs have been observed with a redshift greater than 8.
10 (See
Figure 1.) This causes big problems for cosmologists. First, they believe that GRBs occur at the end of the stellar life-cycle, but as a cosmological redshift, z=8.2 would have the star dying only 800 million years after the Big Bang, when stars shouldn't have even begun to form. Second, at such a great distance, the power would have to be incredible to produce the observed luminosity. This forces cosmologists to think in terms of beaming mechanisms, though the physics involved remains unidentified. The wide range of redshifts, and the total power involved, become much more manageable if the redshift is being modulated by relative velocities at the source. The centroid of a newly forming star might not be moving relative to us, but if the dusty plasma is imploding at 86% of the speed of light, its Doppler shift, interpreted as a cosmological redshift, will put the star at the edge of the known Universe, and at the dawn of time, when it might have actually been within our local group, and the power might not have been anything more than what we would expect out of a normal star.
We can also get an idea for how long it took for the dusty plasma to implode. To figure it as the result of a constant acceleration, we'll neglect the exponentially increasing forces near the end, and just use a straight-line average of the forces during the first 4/5 of the implosion (i.e., where the cell-to-cell spacing was between 10 and 2 meters, as calculated in the
Accretion section), which works out to
7.19 × 1016 m/s
2.
This was with the force in excess of three orders of magnitude greater than gravity. Left up to gravity alone, it would have taken a lot longer. Note that the text above states that stars shouldn't have been forming just 800 million years after the Big Bang, meaning that something is wrong, either with the model of GRBs, and/or with the BBT, while the last calcs showed that dusty plasma can implode in 159 million years. This is true — assuming that there is a supernova to create the Debye cells, and then to strip the sheaths off of them. Without the ionizing radiation, it would have taken a lot longer, which means that the BBT still can't explain stars forming 800 million years after the supposed Big Bang.