© Charles Chandler

 

We know that for the electric force to have the influence described in the previous section, the top layer has to be charged.

 

We can also deduce with confidence that there has to be a strong field between it and an underlying layer. If the Sun only had one charge (positive or negative), the Coulomb force would simply add to the hydrostatic pressure, somewhat more vigorously, and the density would thin out over a much greater distance. The only way to get densely packed plasma that suddenly stops at its outer extent is with an opposite charge below that is pulling it down forcefully. Hence there have to be "current-free double-layers" (CFDLs), where opposite charges cling to each other, but something is preventing recombination.

 

CFDLs wouldn't seem possible in 6,000 K hydrogen, due to its excellent conductivity. But there are two known forces that can keep electric charges separate in the absence of electrical resistance. They are (obviously) the two other forces present at the macroscopic level: the magnetic force, and gravity. We already ruled out magnetism, so we'll investigate the effects of gravity.

 

Figure 1. Charge Separation Under Pressure At left is an abstract representation of a normal crystal lattice, using the familiar icon for an atom, and showing connections of electron shells. At right, extreme pressure has forced the expulsion of one of the electrons from the atom in the center. The expelled electron is to be found just outside, wherever there is room for it, while attracted to the positive ion by the electric force.

It is well-known that at high pressures, matter gets ionized.¹ This is because of the Pauli Exclusion Principle, whereby no two identical fermions (i.e., particles with half-integer spins, such as electrons) may simultaneously occupy the same quantum state in the same location. If atoms are pressed too close together, the electron shells of neighboring atoms overlap, and the conflict forces the liberation of one of the electrons. The extra force required to do this accounts for the incompressibility of liquids and solids.^2,3,4 (See Figure 1.)

 

The same is true even for supercritical fluids — even though they're too hot for crystal lattices, and thus have a plasticity uncharacteristic of their subcritical solid regimes, once compressed down to the density of a solid, they become just as incompressible as subcritical solids.^5,6 This fact isn't salient in the larger body of studies that have been done on supercritical fluids at less extreme pressures, and there's actually one place in the phase diagram where supercritical fluids are compressible beyond even the ideal gas laws — just above the critical temperature. (See the Nelson-Obert charts, and the closely spaced density lines just above the CP in Figure 2.) Nevertheless, the fluid is as incompressible as ever once it achieves its solid density. (See Figure 3.) CO₂ at its critical temperature of 31 °C, when subjected to 300 GPa (i.e., 3 million bars), has a density of 1400 kg/m³, which isn't much above its solid density below the triple point. So supercritical fluids still have a modulus of elasticity beginning at the solid density, but that isn't what we'd call compressibility, certainly not in line with the ideal gas laws.

 

 

Figure 2. Carbon dioxide phase diagram. © 2000 FutureChem

Figure 3. Another representation of the density of the various states.7 In the solid regime, increasing pressure doesn't further compress the matter — it simply enables the closest packed arrangement even at increasing temperatures.

 

 

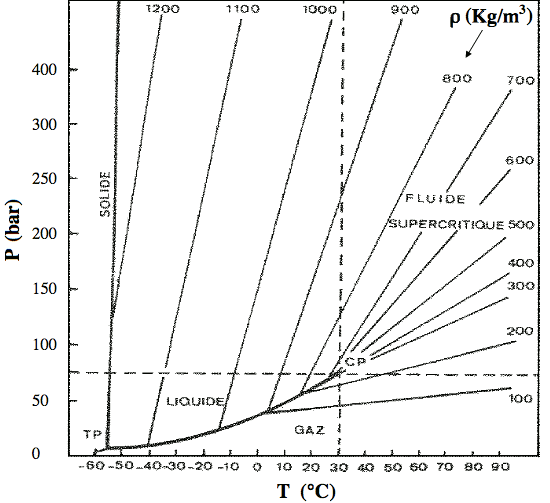
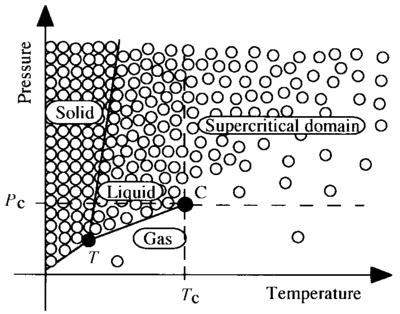
Electron Degeneracy Pressure $$P=\frac{h^2}{20m_em_p^{5/3}}\left(\frac{3}{\pi}\right)^{2/3}\left(\frac{\rho}{\mu_e}\right)^{5/3}$$ where: P = pressure h = Planck's constant me = mass of electron mp = mass of proton ρ = density μe = electron/proton ratio

Thus the Pauli Exclusion Principle holds even for supercritical fluids. Monoatomic matter above the critical point isn't constrained by covalent bonds. But when two atoms are forcibly pushed together, the first conflict will be between the outer electrons. If the pressure is greater than the ionization potential of the atoms, the conflict results in the expulsion of electrons, leaving a strong electrostatic repulsion between the positively charged atomic nuclei, and further compression has to fight the Coulomb force. This marks the transition from compressibility to elasticity, which is very different. The Quantum Mechanics term for this effect is electron degeneracy pressure (EDP), though the Pauli Exclusion Principle predated QM, and is not reliant on it.

 

If the pressure is coming from gravitational loading, it increases with depth, meaning greater compression, and atoms packed closer together. At the threshold for EDP, electrons are expelled, and they have nowhere to go but up, where they will find room between atoms that aren't as tightly packed. Thus the expelled electrons are forced to a higher altitude, leaving positive ions below.

 

The implication not typically considered is that a charge separation has occurred, creating current-free double-layers (CFDLs) — they are layers of opposite charges, with a powerful electric field between them, but there is no current responding to the field, because something prevents it. Usually a sustained charge separation requires an insulator, but in this case, EDP separates the charges and keeps them separate. And gravity is forcing the EDP. So as long as those forces are present, there will be a charge separation, and an electric field.

 

 

So we need to work out the implications of CFDLs inside the Sun.

 

The inner layer is positive, due to the expulsion of electrons under extreme pressure. The liberated electrons congregate at a higher altitude. But that isn't the end of it. The negative layer so produced might go on to induce a positive charge in the layer above it, which will likewise be a CFDL, still in the presence of excellent conductivity. The positive double-layer will be attracted to the negative layer, but repelled by the positive layer below that (i.e., the one created by EDP), and all three will be stable in a positive-negative-positive (PNP) configuration. Such layers created simply by induction can continue ad infinitum, though in spherical layers, the charge density relaxes with each inversion. At some point away from the primary charge separation, the next induced double-layer will not be bound firmly enough to stay organized.

 

So we have deduced with confidence the following facts.

• The electric force is responsible for the extreme density of the photosphere compared to the chromosphere.
• The photosphere is electrically charged.
• There is at least one other layer below it, with the opposite charge, supplying the force necessary to compress the photosphere far beyond the expectations of the ideal gas laws.
• The primary charge separation mechanism is electron degeneracy pressure (EDP), setting up the first two current-free double-layers (CFDLs). Additional layers might also be caused by induction.

We can also deduce the sign of the photosphere's charge, and the relative strength of its charge compared to the underlying layer. There are six possible configurations. There are two possible stacking orders (positive over negative, or negative over positive). Then there are three variations for the relative strengths of the charges (top layer is stronger, underlying layer is stronger, or the charges are perfectly matched).

 

We can dismiss the possibility that the top layer has more charge, since the excess charge would simply drift away.

 

We can also dismiss the possibility that the charges are evenly matched. In CFDLs, the electric field between the layers is greatest at the boundary between them. Moving away from the boundary, the field density diminishes, because of the increased distance from the opposite charge, and because of repulsion from like charges in the same layer. (See Figure 4.) Analogously, in a heavy element, the outer electrons are loosely bound, because of distance from the nucleus, and because of repulsion from electrons in inner shells. The same is true of plasma double-layers. The significance is that with equally matched charges in the solar double-layers, the density of the top layer would still relax gradually to nothing at some distance away. So the distinct limb proves that the underlying charge has to be more powerful, and the top layer has only its densest component. (See Figure 5.)

 

 

Figure 4. Evenly matched charges.

Figure 5. Lower layer more powerful than upper.

 

 

This leaves only two possible configurations, depending on the stacking order (positive over negative, or negative over positive).

 

First we'll consider that the underlying layer is positive. If so, it would easily strip all of the excess electrons from the overlying layer, since they would all be unbound at 6,000 K. Neutral atoms left behind would form a gravitational gradient, tapering off to nothing at infinity. So the underlying layer cannot be positive.

 

The only remaining possibility is that the underlying layer is negative. As such, it will attract positive ions, and ionize neutral atoms to pull in the positive charges that it wants. Excess electrons above such a layer will be repelled by the net negative charge, and thus will not obstruct our view. Hence the distinct limb reveals the extent of a positive double-layer being held down tightly to a far stronger negative layer. The heavy +ions then support hydrodynamic behaviors, where momentum is a considerable factor, and which wouldn't be if the surface was negatively charged.

 

If the surface is positive, held down to an underlying negative layer, and if the driving charge separation mechanism is EDP (which produces a positive layer with an overlying negative layer), there have to be at least three layers. EDP creates a lower body of positive ions, with the expelled electrons forming a negative layer above that, and then there is a positive double-layer around the outside, whose charges were simply induced by the proximity to a negative layer.

 

Figure 6. Convective zone layers. Red = negative; green = positive. Dimensions are in Mm.

Figure 6 depicts this charge configuration. For reasons presented in the next section, these three layers occur entirely within the convective zone, with the degenerate layer being the lower 84 Mm, topped by an electron-rich 105 Mm layer in the middle, and with a 20 Mm induced positive layer at the top.

 

Note that the extra force coming from EDP, and the resultant charge separation, provides an explanation for recent high-precision measurements that revealed that the Sun is not as oblate as it should be.⁸ The equatorial velocity (~2 km/s) should produce a centrifugal bulge, but it doesn't. There is no possible solution to this using Newtonian mechanics. If we asserted that the plasma near the surface was heavier than in the standard model, it would be held down more forcefully by gravity, but it would also have more inertial force, meaning a corresponding increase in centrifugal force, and the bulge would still be there. Somehow, the centripetal force is being increased, without an increase in centrifugal force. This can only be proof of a force that does not vary with mass, and which can only be the electric force. This only makes sense in a model based on CFDLs.

 

Hence by fully processing a few simple facts, we gain a lot of information about the structure of the Sun, at least near the surface. This begs the obvious question of why such reasoning has not been considered before. If it has, it was surely rejected because of the implications. The principles of degenerate matter don't just modify the density gradient at the surface — they deterministically dictate different densities throughout the Sun, especially in the core. Full consideration of those implications leads to a totally new model of the solar interior, with a radically different energy source. This is a bit much for established scientists who have already made a name for themselves within the existing paradigm. But if the data mandate it, this is the road that we must choose, because all other roads will eventually end in impasses.

 

EDP isn't the Pannekoek-Rosseland field.

Re: Interior: Charge separation