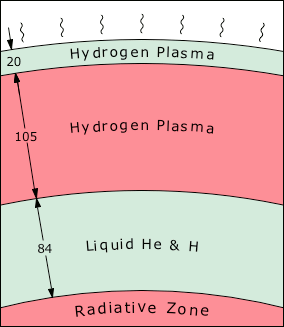
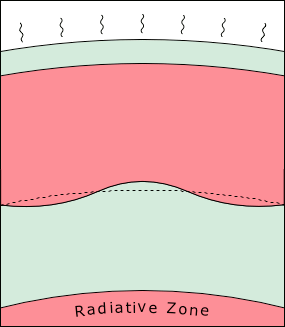
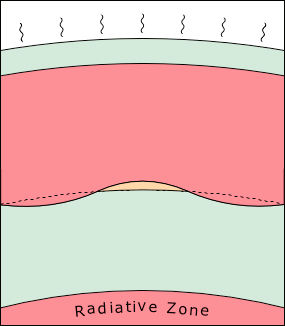
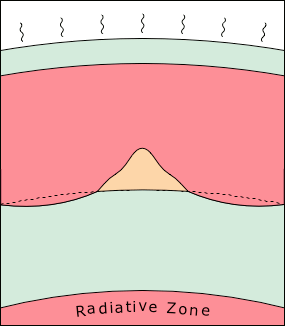
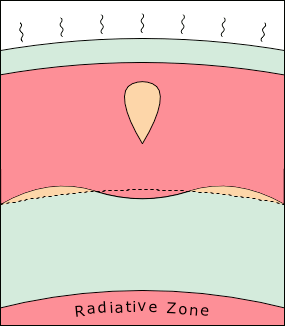
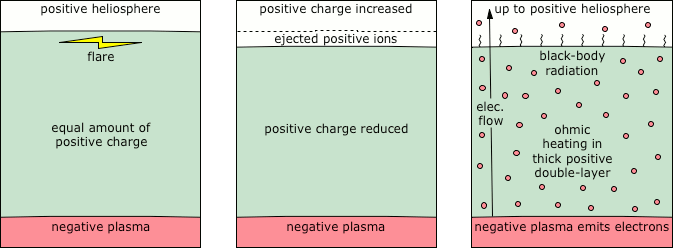
© Charles Chandler

 

The next step is to identify where, exactly, the electrostatic potentials in the Sun are getting discharged. Then we'll estimate the power in those discharges, and see if it matches the known 3.86 × 10²⁶ watts of output from the Sun.

 

The first observable evidence of a solar heat source that we'll consider is the supergranules. These are thermal bubbles that rise at ~.4 km/s, and are typically 30 Mm across. Their nature is poorly understood, but we can get a rough idea of their origins just by their dimensions. The width of a thermal bubble is a function of the depth from which it originates, because during its ascent, smaller bubbles merge into larger ones that rise with less friction. Typically a bubble traverses a depth that is 4 times its width. So if the supergranules are 30 Mm wide, we can guess that they originate from 120 Mm below the surface. And what is going on at that depth? In Figure 1 we can see that at roughly .83 R_⊙ the pressure is sufficient to compress hydrogen into a liquid. This works out to 125 Mm below the surface. So the supergranules originate at the transition from plasma to a supercritical fluid. (See Figure 2.)

 

Figure 2. Convective zone layers. Red = negative; green = positive. Dimensions are in Mm.

Why would such a transition be a source of heat?

 

In the Potentials section we noted that whenever an element is compressed beyond its liquid density, electron degeneracy pressure (EDP) begins to separate the charges. So in addition to the state change at the liquid threshold, there is also a difference in electric charge. Below the threshold, the supercritical fluid is positively charged. Above it, the plasma is negatively charged, since that is where the expelled electrons accumulate. Across this threshold, the electrostatic potential will be enormous. So the release of that potential is the supergranular heat source.^1,2:§5.3

 

All other factors being the same, this gravitational charge separation should be stable, and none of the potential will be discharged. The electrons were expelled from the supercritical fluid because there wasn't the room for them between the atoms. If the pressure doesn't change, the charge separation will not change.

 

The corollary is that electrostatic discharges under these conditions will be triggered if the pressure does change. Since supergranules are evidence of a heat source at roughly the depth of the plasma~liquid boundary, it appears that something is, in fact, altering the pressure. It certainly isn't fluctuations in the gravitational field. But waves (G, P, or S) inside the Sun cyclically alter the pressure. Such alterations occurring precisely at the threshold for EDP will alternately ionize and de-ionize matter. The de-ionization (i.e., electrostatic discharges) will produce heat.

 

Of the types of waves that could make the pressure fluctuate, s-waves are the most interesting, as only they can explain the full complement of characteristics associated with supergranules. First, they have the ability to generate electrostatic discharges, because the crests and troughs of the waves repeatedly cross the threshold for EDP. Above the line, charges can recombine. Below the line, charges are separated again. This generates an alternating current, where ohmic heating initiates thermal bubbles. (See Figure 3, Figure 4, Figure 5, and Figure 6.)

 

 

Figure 3. S-waves at the liquid line elevate the supercritical fluid above the threshold for plasma.

Figure 4. The reduction in pressure allows electron uptake, generating heat.

 

 

Figure 5. The heated plasma forms into a thermal bubble.

Figure 6. Thermal bubble pushes down the next trough.

 

 

Figure 7. Artist's conception of the pattern of supergranules moving across the Sun, courtesy NASA.

Second, s-waves are the only type of wave that can produce the distinctive pattern in which supergranules occur. Rather than popping up randomly across the surface, a line of them progresses across the surface of the Sun.^3,4 The pattern is the most pronounced at the equator.⁵ (See Figure 7.) If the origin of the supergranules is the liquid line (at a depth of 125 Mm), and if they occur in a wave-like pattern, there have to be transverse waves (i.e., s-waves) at the liquid-plasma boundary. Gravity and pressure waves would not produce this pattern. S-waves also explain differential rotation (detailed in the Cycles section), which cannot be explained any other way.

 

So how much heat is brought to the surface by supergranules?

 

Recent research has found that convection transports less than 1/20 of the Sun's total thermal energy to the surface.⁶ So the supergranules themselves are relatively insignificant. It's possible that some of the heat generated at the liquid line is conducted, rather than convected, to the surface. The two transport mechanisms combined might be responsible for as much as 1/6 of the total. But this leaves us still in search of the primary electrostatic discharges.

 

Figure 8. Effects of flashes at different altitudes.

At the surface, we can see arc discharges directly, in the form of solar flares. The heat generated by these discharges is insignificant, but flares can have an interesting side-effect. A flare that occurs above the surface is just a big spark that flashes through a near-perfect vacuum. A flare deep within the Sun, such as one at the liquid line, creates a p-wave, but the impact is fully absorbed by the overlying plasma. But a flare just below the surface creates a p-wave that accelerates the overlying plasma out into space in what is known as a coronal mass ejection (CME). (See Figure 8.) The entire process is complex, and is treated in greater detail in the CMEs section. The amount of power in the flare itself is trivial compared to the overall energy budget, but there is a hidden significance to CMEs within this EM framework.

 

At the bottom of the convective zone, the hydrogen and helium has been compressed into a liquid, and ionized, so it is positively charged. Above the liquid threshold, electrons expelled from the supercritical fluid will congregate, making the plasma negatively charged. Both of those charges should be equally matched, with a powerful electric force pulling them together. Then, on the outside of the negative layer, there will be a positive double-layer. This is because positive ions in the vicinity will be attracted to the negative layer, though repelled by the underlying positive layer, yet the negative layer is closer. Since the electric force obeys the inverse square law, the net force will attract positive ions and repel electrons, and a layer of positive charge will build up on the outside of the negative layer. For reasons presented later, the depth of this layer is estimated at 20 Mm.

 

The significance is that CMEs near the surface are occurring in a positive double-layer, and the ejections affect a net loss of positive charge. This leaves the Sun with a net negative charge, and creates an electrostatic potential between the Sun and the heliosphere.^7,8 This solar~heliospheric electric field is not powerful enough to create arc discharges in the atmosphere. But it can still motivate an electric current, and therefore generate ohmic heating. (See Figure 9.)

 

 

Figure 9. The depletion of the positive double-layer by a flare motivates a flow of electrons, from the negative layer out into the heliosphere. The depletion is greatly exaggerated in the images. One CME reduces the overall radius of the Sun by a mere 10⁻¹⁰ m.

 

 

How much ohmic heating?

 

We know the average mass of a CME, and the rate at which they occur. All we have to do is assign a positive charge to all of that mass, and find the net loss of positive charge. The equal-but-opposite reaction will be a subsequent electron drift through the positive layer. Knowing the voltage,^9:6,10 we can calculate the watts from ohmic heating, and compare that to the known power output of the Sun.

 

The number of CMEs observed per day on the side of the Sun facing us ranges from .2 at the minimum to 3.5 at the maximum, for an average of 1.85 per day. So we just double that number, to account for the CMEs on the opposite side of the Sun, for an average of 3.7 CMEs per day.

mass loss to CMEs	=	CMEs/day	×	mass/CME
	=	3.7 CME/day	×	1.60 × 1012 kg/CME
	=	5.92 × 1012 kg/day
	=	6.85 × 107 kg/s

Estimates for the total solar mass loss are two orders of magnitude higher, ranging from 1.38 × 10⁹ to 5.76 × 10⁹ kg/s.^11:3 The discrepancy is likely due to microflares and spicules that expel matter, which do not qualify as CMEs per se, but which nevertheless have the same effect on the balance of charges in the Sun. To account for this while still being conservative, we can simply bump up the number based on CMEs by one order of magnitude, making it 6.85 × 10⁸ kg/s. So if one tenth of the atoms expelled from the Sun are +ions, the following calculations will be reasonable.

 

Assuming that all of this is hydrogen, we can find the rate at which atoms are expelled.

atoms expelled per second	=	rate of expulsion	×	atoms/kg of hydrogen
	=	6.85 × 108 kg/s	×	5.98 × 1026 atoms/kg
	=	4.10 × 1035 atoms/second

Now we can find the "current" (in +ions) that will drive the equal-but-opposite drift of electrons.

current due to +ion loss	=	rate of +ion expulsion	×	charge per +ion
	=	4.10 × 1035 atoms/second	×	1.60 × 10−19 Coulombs/atom
	=	6.56 × 1016 Coulombs/second
	=	6.56 × 1016 A

Knowing the amps and the volts, we can find the watts.

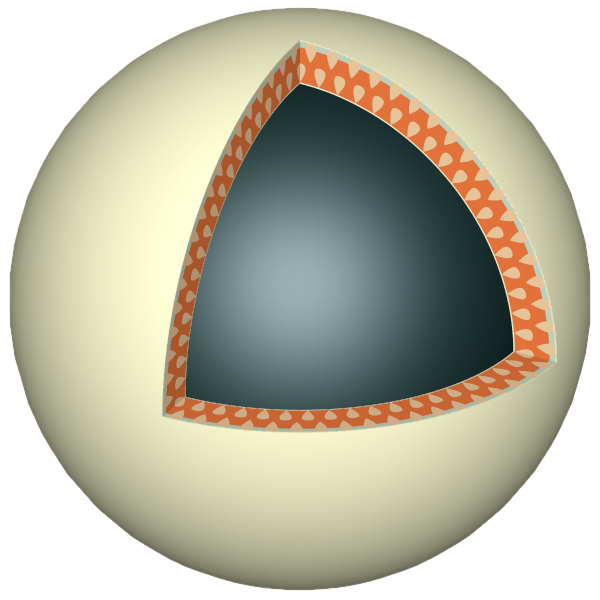
watts	=	amps	×	volts
	=	6.56 × 1016 A	×	1.70 × 109 V
	=	1.12 × 1026 W

The known power output of the Sun is 3.86 × 10²⁶ W.^{12:980-985,11:3} Assuming that 1/3 of the power is coming from nuclear fusion (1.29 × 10²⁶ W), 1/6 is coming from the arc discharges that motivate the supergranules (6.43 × 10²⁵ W), and 1/2 is coming from near-surface ohmic heating as just calculated (1.12 × 10²⁶ W), the total is 3.05 × 10²⁶ W, which is very close to the observed 3.86 × 10²⁶ W. Thus the solar energy budget has been balanced, and without having to alter subatomic theory to make the "neutrino problem" go away.

 

So what causes the nuclear fusion that provides 1/3 of the power? If there isn't any fusion in the core, there isn't any fusion at all just due to extreme pressures from gravity. So what else could cause nuclear fusion?

 

The answer is arc discharges. Precursors for fusion have been found in lightning strikes here on Earth.¹³ This is believed to occur at the ends of the discharge channels, where relativistic electrons slam into the STP gas, instantaneously creating the necessary temperatures and pressures. Note that the only "plasma confinement" mechanism is the inertial forces of the gas itself, but as the channel advances in stepped leaders, the hard x-rays (and sometimes even gamma rays) are distinctive, and lingering free neutrons have been detected. (So this is a type of "inertial confinement fusion," though it is very different from nuclear energy research.) On Earth, the discharge channels are only ~5 km long, with stepped leaders 100 m long. The discharges in the Sun can be over 100 Mm long, and the electrons are accelerated to nearly the speed of light. Evidence of fusion directly associated with solar flares has been confirmed.^14,15 Since the pressure in the convective zone is nothing compared to the requirements for fusion, this is the only possible set of conditions that could produce it.

 

With this in mind, it makes sense that fusion accounts for 1/3 of the total energy, and charge recombination accounts for the other 2/3. If it takes an arc discharge to cause fusion, the discharge itself produces some energy, and if this didn't show up in the budget, something would be wrong.

 

So in the most fundamental sense, the prime mover is the electric force. The "like-likes-like" principle (as described in the Accretion section) pulled matter together to create the Sun out of a dusty plasma. EDP created alternating layers of positive and negative charges, adding the force necessary to keep the final aggregate organized. The electrostatic potentials between these layers are being slowly converted to kinetic energy as arc discharges expel material, and as the resulting electrostatic imbalance causes a steady electric current. The discharges also produce the conditions for fusion. Without the electric force, none of this would have happened.

 

 

Figure 10. The layers responsible for the heat & light released by the Sun. Positively charged liquid hydrogen (green) is topped by negatively charged plasma (dark orange), with a positive double-layer (yellow) at the very top. S-waves in the liquid hydrogen generate supergranules (light orange). The solar~heliospheric current through the positive double-layer generates the ohmic heating that is responsible for 1/2 of the blackbody radiation.

 

 

To summarize, there are no identified energy sources below 125 Mm. Nuclear fusion in the core is not possible, and there is no evidence of electrostatic discharges below the liquid line. Hence the "radiative zone" doesn't radiate anything. (Where the term is used herein, it only designates the mid-region of the Sun's interior, which we know to be there from helioseismology. It gets its name from the role that it plays in the "fusion furnace" model, while in the present model, the name is a misnomer.) For that matter, only the upper half of the "convective zone" actually convects, and even then, convection is responsible for less than 1/20 of the heat transported to the surface.⁶ In the present model, as much as 1/2 of the total heat is generated at the liquid line (1/6 by arc discharges and 1/3 by nuclear fusion). Most of this heat is transported to the surface by conduction in the supercritical plasma. The remaining 1/2 of the heat is generated in the topmost 20 Mm by ohmic heating.

 

Calculations