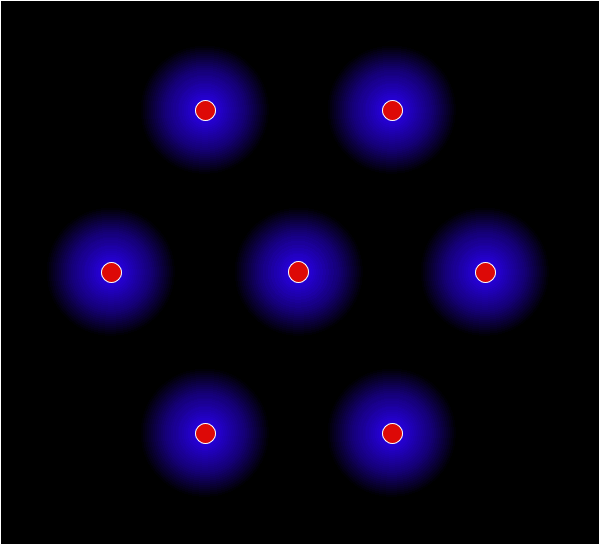
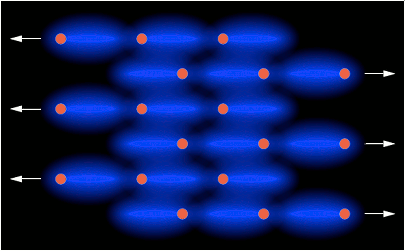
© Charles Chandler

 

There is an organizing principle in physics that operates across a broad range of scales, from the force that binds atoms together into molecules, to the force that organizes stars into galaxies — it's the electric force.

 

Figure 1. Electric forces between a neutrally charged helium atom at left, with 2 electrons as point sources at the K shell radius of 31 pm, and a positive test charge at right, arbitrarily 310 pm from from the helium nucleus, showing a slight net attraction.¹ At the same distances, gravity is just 7.77 × 10⁻⁴⁵ N, so it isn't a factor.

Richard Feynman was the first to realize that there is a hidden significance to the electric force's inverse square law that is important in bringing atoms together to make molecules.^{2:ch2:pg2,3,4,5,6,7} He said that it requires ionization, because a positive ion is attracted to a neutrally charged atom. The reason is that the negative charges in the neutral atom's electron cloud get nearer to the distant positive ion. Then if we just look at the effects of the inverse square law, we realize that the near-side electrons exert a greater attraction per elementary charge on the positive ion than the repulsion from the atomic nucleus, and the attraction to the far-side electrons isn't that much less. If we add up all of these +/− forces, we find that the neutral atom shows a slight net negative charge at a distance. (See Figure 1.)

 

This means that any nearby positive ion will be pulled toward the neutral atom, despite the net charge of the whole assembly being positive (ergo the paradoxical "like-likes-like" effect). We should also note that the attraction gets stronger as the positive ion gets nearer to the neutral atom, since the relative proximity of the positive ion to the near-side electrons gets more dramatic. It's also possible (depending on which atomic model is used) that the electrons favor that near side, being attracted to the positive ion. If there is even more negative charge on the near side of the neutral atom, the positive ion will experience an even greater attraction. So the positive ion is pulled toward the neutral atom, to the point of sharing electrons with it, thereby forming a molecule.

 

The corollary is that if both atoms are neutrally charged, they will both be showing a net negative charge at a distance, and they will repel each other. This is why the elements with fully populated outer electron shells (i.e., the noble gases) are the least likely to form molecules — electrostatic repulsion within the shells calls for an equal distribution of electrons, and if the shell is fully populated, there is nowhere for electrons to go if exposed to a negative test charge at a distance, so they stay where they were, and push back. This is also why the elements with the lowest ionization potentials are the best at forming molecules — once one of the atoms gets ionized, the net electric force goes from repulsive to attractive.*¹⁴⁵³²

 

Figure 2. The charges within atoms get polarized in an external electric field, producing an attraction between them.

A related phenomenon is the molecule-building facilitated by an external electric field, which chases electrons to the far sides of their atoms, making electric dipoles out of the atoms. With the negative side of one atom facing the positive side of the other, there is an electrostatic attraction between the atoms. (See Figure 2.) This can turn a gas into a liquid, a liquid into a liquid crystal,^3,4,5,6 or a gas into a polymerized solid.

 

This same principle also acts on an astronomical scale. In the beginning, nothing existed except huge clouds of weakly ionized gas & dust, known as dusty plasmas. Somehow, these extremely diffuse clouds collapsed into very dense stars, planets, & moons, but Newtonian physics can't explain how this ever could have happened. Gravity will pull the matter inward, but if it succeeds in compressing the gas, the hydrostatic pressure inside the gas will push back out. As the compression continues, the outward force of internal pressure will increase faster than the inward force of gravity, meaning that eventually, the pressure will be as strong as gravity. Once the equilibrium has been achieved, further compression can't happen, because there isn't any net force. For example, the Earth's atmosphere is far cooler than a dusty plasma if compressed down to the same density,⁸ and our atmosphere is under the Earth's gravity, which is a lot stronger than the gravity inside a dusty plasma with nothing else around, and yet the air doesn't collapse under its own weight, because the hydrostatic pressure won't let it.

 

Such being the case, it's no surprise that modern telescopes have detected primordial plasmas that refuse to collapse, some of which seeming to have been around since the beginning of time. If they were going to collapse under their own weight, they already would have. If they haven't already, it's because gravity alone isn't enough — there has to be another force.

 

Studying the conditions in which a dusty plasma collapses reveals the nature of that force. The dusty plasma has to be hit by the ejecta from a supernova, or it has to collide with another dusty plasma. What's the significance? It isn't that the collapse has been facilitated by a stronger gravitational attraction in denser matter, because there will also be an increase in hydrostatic pressure that will more than offset the gravity. Furthermore, the combined velocities in such collisions are typically above 20 km/s, and the thermalization of hypersonic collisions should greatly increase the hydrostatic pressure, causing the expansion (or even the explosion) of the mixture.

 

Figure 3. Resting dusty plasma, made up of Debye cells that repel each other.

Figure 4. Debye sheaths stretched into comas by ram pressure stripping.

So we have to look at non-Newtonian effects of dusty plasma collisions that might generate attractive body forces. And we won't have to look very far. Dusty plasmas are made up of Debye cells, with negatively charged nuclei, and positively charged sheaths. (See Figure 3.) This is the same electrostatic configuration as atoms surrounded by electron clouds, except the polarity is inverted. Still, the electric force works the same either way, and in the resting condition, net-neutral Debye cells repel each other. At a typical spacing of 10 m (center to center), and with just 1 charged particle in 10¹⁵ neutrals, the electrostatic repulsion between the Debye sheaths is stronger than the gravitational attraction.⁹ This means that hydrostatic pressure isn't the only reason dusty plasmas don't collapse under their own weight — long before the cells even come into contact with each other and start building up pressure, the electric force offsets gravity, and the compression never even begins. Thus they are noble dusty plasmas, so to say, with "fully populated" Debye sheaths, and all of them are showing a net positive charge at a distance, meaning a net repulsion between them. This is what led Irving Langmuir to label them as "plasmas" as an analogy for blood cells that insulate their contents from their neighbors.¹⁰

 

But look at what happens in Figure 4, where two sets of Debye cells collide — the sheaths get stretched into comas by the friction. And this is the same electrostatic configuration as Figure 2, where polarization of particles resulted in an attractive force that brought everything together. So in this form, the net electric force is attractive, and now it's 3 orders of magnitude stronger than gravity.⁹ And just like it was with atoms, the closer the cells get to each other, the greater the attraction, because of the inverse square law. Now the dusty plasma is definitely going to implode into a star.

 

Interestingly, supernovae are better triggers of star formation than simple gas cloud collisions. In both cases, large bodies of Debye cells collide, with the effects already described. But what's different about supernovae? In addition to the ejecta, they also generate large batches of UV radiation. This photo-ionizes the matter, enabling atoms to form into molecules, as Feynman noted. Likewise the molecules can condense into particles, and then Debye cells can form. All other factors being the same, these would be resting Debye cells, as in Figure 3, which repel each other. And the greater the degree of ionization, the greater the repulsion. But then the ejecta from the supernova arrive, stretching the Debye sheaths into comas, and generating an electrostatic attraction that causes the cells to collapse into a star. So it's the same "like-likes-like" principle at two different scales, and supernovae just happen to provide the catalysts for both processes.

 

Figure 5. NGC 1427A

Figure 6. ESO 325-G004

And there is yet another scale at which the same principle operates — that of galaxies. Over 6 billion years ago, most of the galaxies were irregular clumps of stars, such as in Figure 5. Now most galaxies are radially symmetrical, such as in Figure 6.¹¹ Somehow, all of the stars are getting coerced into a regular form. This appears to be the consequence of galactic mergers.^12:12/22/23 But that doesn't explain the regularity of the result — the shape of the merger should be even more complex than the components.

 

One possibility is that somehow, the merger forced the implosion of the galaxy. No matter how irregular the assembly, there will always be a centroid, which will be the focal point of any implosion. And the ejecta from the resulting explosion will always be radially symmetrical. With a little bit of angular momentum, the resulting galaxy will be elliptical, while a lot will exaggerate the aspect ratio into a disc. Either way, in just one action, the most peculiar of shapes can be morphed into near-perfect symmetry. But why would the merger of two or more smaller galaxies result in the implosion of the entire assembly? All other factors being the same, we'd expect the components to already be in hydrostatic equilibrium. The combination would then have twice the mass, and thus more gravity, but it will also have twice the hydrostatic pressure, plus the thermalization of the combined velocities. So just like with colliding dusty plasmas, we'd expect the collision of two or more galaxies to blow them apart, not cause them to implode on each other. But therein lies the answer, because just like dusty plasmas, stellar systems are charged bodies surrounded by plasma sheaths. If our Sun were involved in such a collision, our spherical heliosphere would be stretched into a coma by friction with other heliospheres or with interstellar plasmas. Since the heliosphere has a slight positive charge,^13,14 and assuming that other stars have a similar set-up, we have the same configuration as in Figure 4, where there will be an electrostatic attraction between stars far stronger than gravity. Now the stellar systems are going to implode on the galactic centroid. The only difference is that they aren't going to get compressed into one galactic star — there are limits to how big a star can get before a Type 1a supernova blows it apart. So the implosion results in a violent explosion, sending all of the matter hurling back out — and in a radially symmetrical form.

 

Thus the electric force is the organizing principle of the Universe, and interestingly, it seems that the same configuration does the job at several different scales, pulling atoms together into molecules, molecules into particles, particles into Debye cells, Debye cells into stars, and stars into galaxies — all on the basis of a mutual attraction to a shared opposite. Recent research suggests that it might even be the force holding together the nuclei of atoms,¹⁵ so this needs to be further investigated.